Question

2.What is the most precise name for quadrilateral ABCD with vertices A(-2,4), B(5,6), C(12,4), and D(5,2)?<br />

A. parallelogram<br />
B. rhombus<br />
C. quadrilateral<br />
D. rectangle<br /><br />
3. What is the most precise term for quadrilateral ABCD with vertices A(1,2), B(2,6), C(5,6), and D(5,3)?<br />
A. square<br />
B. rhombus<br />
C. parallelogram<br />
D. kite<br /><br />
9. What is the measure of one angle in a regular 16-gon?<br />
A. 2,520 <br />
B. 202.5 <br />
C. 157.5<br />
D. 78.75<br /><br />
10. The measure of exterior angle of a regular 5-gon is ____ the measure of each exterior angle of a regular 9-gon?<br />
A. greater than<br />
B. less than<br />
C. equal to

Answer 1

A / admiration

Explanation:

c

Answer 2

Answer: The correct options are (2). A, (3). B, (9). C and (10). A.

Step-by-step explanation: The calculations are as follows:

(2) The vertices of the quadrilateral ABCD are A(-2,4), B(5,6), C(12,4), and D(5,2).

The length of the sides are calculated using distance formula as follows:

`AB=√((5+2)^2+(6-4)^2)=√(49+4)=√(53),\\\\BC=√((12-5)^2+(4-6)^2)=√(49+4)=√(53),\\\\CD=√((5-12)^2+(2-4)^2)=√(49+4)=√(53),\\\\DA=√((-2-5)^2+(4-2)^2)=√(49+4)=√(53).`

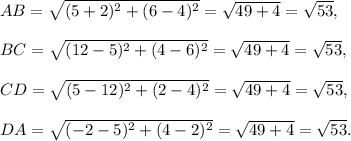
The slopes of the sides are calculated as:

`\textup{Slope of }AB=(6-4)/(5+2)=(2)/(7),\\\\\\\textup{Slope of }BC=(4-6)/(12-5)=-(2)/(7),\\\\\\\textup{Slope of }CD=(2-4)/(5-12)=(2)/(7),\\\\\\\textup{Slope of }DA=(4-2)/(-2-5)=-(2)/(7).`

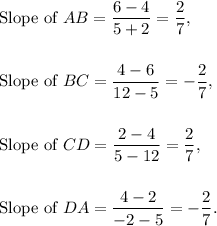
Since the lengths of all sides are equal and the opposite sides are parallel, because the slopes of two parallel lines are equal.

Therefore, ABCD is a parallelogram because opposite sides are parallel and congruent.

Thus, (A) is the correct option.

(3) The vertices of the quadrilateral ABCD are A(1, 2), B(2,6), C(5, 6), and D(5, 3).

The length of the sides are calculated using distance formula as follows:

`AB=√((2-1)^2+(6-2)^2)=√(1+16)=√(17),\\\\BC=√((5-2)^2+(6-6)^2)=3=,\\\\CD=√((5-5)^2+(3-6)^2)=3,\\\\DA=√((1-5)^2+(2-3)^2)=√(16+1)=√(17).`

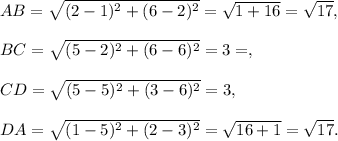
The slopes of the diagonals are

`\textup{Slope of }AC=(6-2)/(5-1)=1,\\\\\\\textup{Slope of }BD=(3-6)/(5-2)=-1.`

So, slope of AC × slope of BD = 1 × (-1) = - 1. Hence the diagonals are perpendicular.

Therefore, ABCD is a kite, because adjacent sides are congruent and diagonals are perpendicular to each other.

Thus, (B) is the correct option.

(9) A 16-gon is a polygon with 16 sides.

So, the measure of one exterior angle of a regular 16-gon is

`E_a=\fracx{360^\circ}{16}=22.5^\circ.`

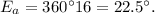
Therefore, the measure of one angle is given by

`I_a=180^\circ-22.5^\circ=157.5^\circ.`

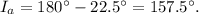
Thus, (C) is the correct option

(10) The measure of exterior angle of a regular 5-gon is

`E_5=(360^\circ)/(5)=72^\circ,`

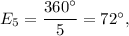
and the measure of each exterior angle of a regular 9-gon is

`E_9=(360^\circ)/(9)=40^\circ,`

Therefore, The measure of exterior angle of a regular 5-gon is greater the measure of each exterior angle of a regular 9-gon.

Thus, (A) is the correct option.

Hence, the correct options are (2). A, (3). B, (9). C and (10). A.