Question

Find the area of quadrilateral ABCD. [Hint: the diagonal divides the quadrilateral into two triangles.]A. 28.53 units²B. 26.47 units²C. 27.28 units²D. 33.08 units²

Answer 1

A. 28.53 units²

Step-by-step explanation

Finding the area of irregular quadrilateral ABCD, we divide the given figure into shapes (two triangles) as shown below:

Then, we find the area of the two triangles.

Triangle ABD:

`\begin{gathered} Area=√(s(s-a)(s-b)(s-c)) \\ \\ s=(a+b+c)/(2)=(2.89+8.59+8.6)/(2)=(20.08)/(2)=10.04 \\ \\ Area\text{ }of\text{ }triangle\text{ }ABD=√(10.04(10.04-2.89)(10.04-8.59)(10.04-8.6)) \\ \\ Area\text{ }of\text{ }triangle\text{ }ABD=√(10.04(7.15)(1.45)(1.44)) \\ \\ Area\text{ }of\text{ }triangle\text{ }ABD=√(149.889) \\ \\ Area\text{ }of\text{ }triangle\text{ }ABD=12.24\text{ }unit^2 \end{gathered}`

Triangle ADC:

`\begin{gathered} Area=√(s(s-a)(s-b)(s-c)) \\ \\ s=(a+b+c)/(2)=(4.3+7.58+8.6)/(2)=(20.48)/(2)=10.24 \\ \\ Area\text{ }of\text{ }triangle\text{ }ADC=√(10.24(10.24-4.3)(10.24-7.58)(10.24-8.6)) \\ \\ Area\text{ }of\text{ }triangle\text{ }ADC=√(10.24(5.94)(2.66)(1.64)) \\ \\ Area\text{ }of\text{ }triangle\text{ }ADC=√(265.346) \\ \\ Area\text{ }of\text{ }triangle\text{ }ADC=16.29\text{ }unit^2 \end{gathered}`

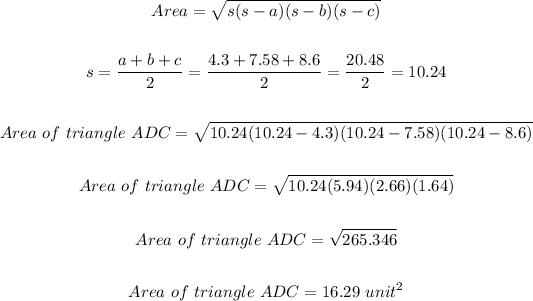
Therefore, the area of the quadrilateral ABCD = the Sum of the two triangles

The area of the quadrilateral ABCD = 12.24 units² + 16.29 units² = 28.53 units²