Answer: Exact solutions are x = 2√2 and x = -2√2
Approximate solutions are x = 2.828 and x = - 2.828
The attachment shows a portion of the graph with this equation. The "zeros" are the solutions.
Step-by-step explanation: To get values for x, we need to isolate x
2x² -6 = 10 Add 6 to both sides and rewrite the equation as
2x² = 16 divide both sides by 2
x² = 8 Factor 8 as 4(2) so you can "pull out" a square.
To solve for x, take the square roots of both sides.
Remember that square roots are positive and negative values.
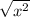 = ±
= ±
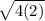
x = ± 2√2
x = 2√2 and x = -2√2
We can use a calculator to get approximate values of x.
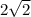 = ±2.828427125
= ±2.828427125
Round that to x = 2.828 or 2.83 AND x = - 2.828 for approximate values.