Answer:
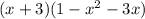
Explanation:
First, let's factor that quadratic on the right:
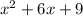 happens to factor nicely into
happens to factor nicely into
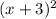 , and putting that into our expression gets us
, and putting that into our expression gets us
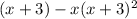
Factoring out an (x + 3) from each term, we get
![(x+3)[1-x(x+3)]](https://img.qammunity.org/2022/formulas/mathematics/high-school/y7kk3i8eu871k39mlj20ws2nxaobydy3ab.png)
Simplifying that expression on the right:
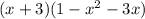
We can't factor that right-side expression any more, so we can just leave it like that!