If two lines are perpendicular, then the product of their slopes is equal to -1.
If two lines are parallel, then their slopes are equal.
Write the equation of the lines P and Q in slope-intercept form by isolating y. Compare their slopes to see if they are either parallel or pependicular, or none.
The equation of a line with slope m and y-intercept b in slope-intercept form, is:

Line P:
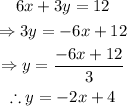
Then, the slope of the line P is -2.
Line Q:
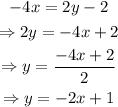
Then, the slope of the line Q is -2.
Since both lines have the same slope, then they are parallel.