Answer: Choice A
========================================================
Step-by-step explanation:
The left side
 simplifies to
simplifies to
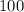 since
since
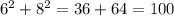
The right side is also 100 because
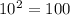
So
 is a true equation because both sides are the same number.
is a true equation because both sides are the same number.
Therefore, a triangle with sides 6,8,10 is a right triangle. The hypotenuse 10 is the longest side opposite the 90 degree angle. In other words, the 90 degree angle is between the sides 6 ft and 8 ft.
So this is why choice A is the final answer.
-----------
Choice B is false because the inequality
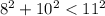 becomes
becomes
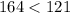 which is also false. Even if that inequality was true, we can't use it to form a right triangle. We need both sides to be equal.
which is also false. Even if that inequality was true, we can't use it to form a right triangle. We need both sides to be equal.
Choice C is false because we need to have both sides equal to the same number. We need to have
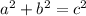 to be true if we want a right triangle with sides a,b,c.
to be true if we want a right triangle with sides a,b,c.
Choice D is false because of the phrasing "Draw any two of the sides with a right angle between them". The 90 degree angle must go between the 6 and the 8, and nowhere else.