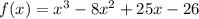Question

Finding a polynomial of a given degree with given zeros: Complex zeros

Answer 1

Given:

• Degree of polynomial = 3

,

• Zeros of the polynomial: 2, 3 - 2i

Let's find the polynomial.

Since the polynomail is of degree 3, it's highest exponent will be 3.

Equate the zeros to zero:

x = 2

Subtract 2 from both sides:

x - 2 = 2 - 2

x - 2 = 0

x = (3 - 2i)

Since this root is a complex conjugate, we have the other complex root: (3 + 2i)

Hence, we have:

(x - (3 - 2i)) and (x - (3 + 2i)).

Therefore, to write the function, we have:

`f(x)=(x-2)(x-(3-2i))(x-(3+2i))`

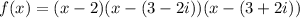
Now, simplify the expression:

`\begin{gathered} f(x)=(x-2)(x-3+2i)(x-3-2i) \\ \\ f(x)=x(x-3+2i)-2(x-3+2i)(x-3-2i) \\ \\ f(x)=x^2-3x+2ix-2x+6-4i(x-3-2i) \\ \\ f(x)=x^2-5x+2ix-4i+6(x-3-2i) \end{gathered}`

Solving further:

`\begin{gathered} f(x)=x(x^2-5x+2ix-4i+6)-3(x^2-5x+2ix-4i+6)-2i(x^2-5x+2ix-4i+6) \\ \\ f(x)=x^3-5x^2+2ix^2-4ix+6x-3x^2+15x-6ix+12i-18-2ix^2+10ix-4i^2x-8-12i^{} \end{gathered}`

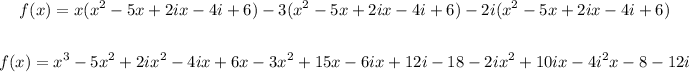
Combine like terms:

`\begin{gathered} f(x)=x^3-5x^2-3x^2-4ix-6ix+10ix+2ix^2-2ix^2+6x+15x+12i-12i-8-16 \\ \\ f(x)=x^3-8x^2+25x-26 \end{gathered}`

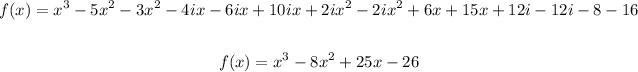
ANSWER:

`f(x)=x^3-8x^2+25x-26`