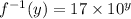Question

I need help question 10 b and c

Answer 1

Part b.

In this case, we have the following function:

`y=5(2.4)^x`

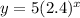
First, we need to solve for x. Then, by applying natural logarithm to both sides, we have

`\log y=\log (5(2.4^x))`

By the properties of the logarithm, it yields

`\log y=\log 5+x\log 2.4`

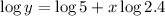
By moving log5 to the left hand side, we have

`\begin{gathered} \log y-\log 5=x\log 2.4 \\ \text{which is equivalent to} \\ \log ((y)/(5))=x\log 2.4 \end{gathered}`

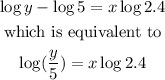
By moving log2.4 to the left hand side, we obtain

`\begin{gathered} (\log(y)/(5))/(\log2.4)=x \\ or\text{ equivalently,} \\ x=(\log(y)/(5))/(\log2.4) \end{gathered}`

Therfore, the answer is

`f^(-1)(y)=(\log(y)/(5))/(\log2.4)`

Part C.

In this case, the given function is

`y=\log _(10)((x)/(17))`

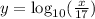
and we need to solve x. Then, by raising both side to the power 10, we have

`\begin{gathered} 10^y=10^{\log _(10)((x)/(17))} \\ \text{which gives} \\ 10^y=(x)/(17) \end{gathered}`

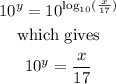
By moving 17 to the left hand side, we get

`\begin{gathered} 17*10^y=x \\ or\text{ equivalently,} \\ x=17*10^y \end{gathered}`

Therefore, the answer is

`f^(-1)(y)=17*10^y`