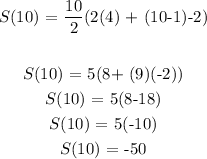Question

a) Consider an arithmetic series 4+2+0+(-2)+.....i) What is the first term? And find the common difference d.ii) Find the sum of the first 10 terms S(10).b) Solve
`{2}^(x - 3) = 7`

Answer 1

Step-by-step explanation:

Here, we want to work with an arithmetic series

a) First term

The first term (a) of the arithmetic is the first number on the left

From the question, we can see that this is 4

Hence, 4 is the first term

To find the common difference, we have this as the difference between twwo subsequent terms, going from left to right

We have this as:

`2-4\text{ = 0-2 = -2-0 = -2}`

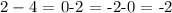
The common difference d is -2

ii) We want to calculate the sum of the first 10 terms

The formula for this is:

`S(n)\text{ = }(n)/(2)(2a\text{ + (n-1)d)}`

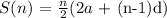
Where S(n) is the sum of n terms

n is the number of terms which is 10

a is the first term of the series which is 4

d is the common difference which is -2

Substituting these values, we have it that:

`\begin{gathered} S(10)\text{ = }(10)/(2)(2(4)\text{ + (10-1)-2)} \\ \\ S(10)\text{ = 5(8+ (9)(-2))} \\ S(10)\text{ = 5(8-18)} \\ S(10)\text{ = 5(-10)} \\ S(10)\text{ = -50} \end{gathered}`