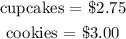Question

All you need is in the photo please answer fastplease

Answer 1

The cost of cupcakes and cookies are;

`\begin{gathered} \text{cupcakes = \$2.75} \\ \text{cookies = \$3.00} \end{gathered}`

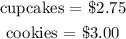
Step-by-step explanation:

Let x and y represent the cost of a cupcake and cookie respectively.

Given that;

Five cupcakes and two cookies cost $19.75.

`5x+2y=19.75-------1`

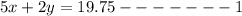
Two cupcakes and four cookies cost $17.50.

`2x+4y=17.50-------2`

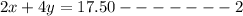
Let's solve the simultaneous equation by elimination;

multiply equation 1 by 2;

`10x+4y=39.50-------3`

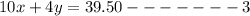
subtract equation 2 from equation 3;

`\begin{gathered} 10x-2x+4y-4y=39.50-17.50 \\ 8x=22 \\ \text{divide both sides by 8;} \\ (8x)/(8)=(22)/(8) \\ x=2.75 \end{gathered}`

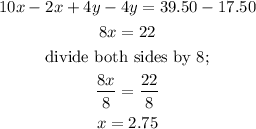
since we have the value of x, let substitute into equation 1 to get y;

`\begin{gathered} 5x+2y=19.75 \\ 5(2.75)+2y=19.75 \\ 13.75+2y=19.75 \\ 2y=19.75-13.75 \\ 2y=6 \\ y=(6)/(2) \\ y=3.00 \end{gathered}`

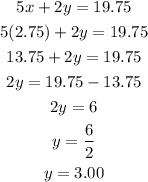
Therefore, the cost of cupcakes and cookies are;

`\begin{gathered} \text{cupcakes = \$2.75} \\ \text{cookies = \$3.00} \end{gathered}`