Question

An equation for the depreciation of a car is given by y = A(1 – r)t , where y = current value of the car, A = original cost, r = rate of depreciation, and t = time, in years. The value of a car is half what it originally cost. The rate of depreciation is 10%. Approximately how old is the car?

Answer 1

Answer: The answer is 6.6 Years for people that don't want to round

Step-by-step explanation: ^^

Answer 2

The car is 6.5788 years old.

Explanation:

The key to solve the problem is in the following sentence: "The value of a car is half what it originally cost". Keep in mind that
`y` is the current value and
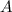`A` is the original cost. It means that
`y` is half of
`A`:
`y=(A)/(2)`.

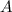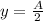It is assumed that the rate of depreciation is annually and its value is
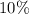`10\%`. Remember that
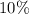`10\%` equals
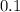`0.1`, so
`r=0.1`.

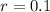For finding the value of
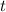`t`, you must replace the values of
`y` and
`r` in the depreciation formula:

`y=A\cdot (1-r)^t`

`(A)/(2)=A\cdot (1-0.1)^t`

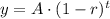
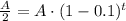
After cancelling the variable
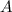`A` the equation would be:

`(1)/(2)=(0.9)^t`

For finding the value of
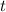`t` you must apply natural algorithm in both sides:

`ln\bigg( (1)/(2)\bigg) =ln[(0.9)^t]`

`ln(0.5)=t\cdot ln(0.9)`

`t=(ln(0.5))/(ln(0.9))`

`t=6.5788`

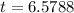
The previous value can be split in two parts:
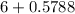`6+0.5788`. The first part refers to years and the second part can be converted to months by multiplying the total months in a year (
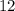`12`) by
`0.5788`.

`months=12* 0.5788=6.9456`

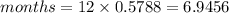
Thus, the car is 6.5788 years old (which is approximately 6 years and almost 7 months).