Question

How would I write an equation in point- slope form with inequalities, slope-intercept form with inequalities and standard form with inequalities with these three sets of points(9,7) (8,5)(2,9) (2,7)(3,5) (5,4)

Answer 1

a. The point-slope equation is:

`y-y_1=m(x-x_1)`

Where m is the slope and (x1,y1) are the coordinates of one point in the line. Also, you need to write the equation with inequalities, then you need to replace the = sign, for a <, > or <=, >= sign.

Let's start by finding the slope of the first set of points (9,7) (8,5).

The formula for the slope is:

`m=\frac{y_2-y_1}{x_2-x_1_{}}`

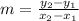
By replacing the values you obtain:

`m=\frac{5-7_{}}{8_{}-9}=(-2)/(-1)=2`

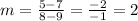
The slope is 2.

Now, replace this value into the slope-form equation and the values of the first point (9,7):

`y-7_{}>2(x-9)`

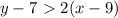
I choose the sign > (greater than), but you can choose anyone, the difference will be for the solution of the inequality. When you solve the inequality you will find that the x-values have to be greater than the solution you found, or less than... etc, it will depend on the sign you have in the inequality.

b. The slope-intercept equation is:

`y=mx+b`

Where m is the slope and b the y-intercept.

Let's use the second set of points (2,9) and (2,7)

Start by calculating the slope:

As there's no difference in the x-coordinates, the line is a vertical line at x=2.

Also, there's no y-intercept as the line never crosses the y-axis.

I will use the first set again, so you can understand the slope-intercept form.

From part a) you know that the slope is 2, let's replace it in the equation and use the first pair of coordinates to find b:

`\begin{gathered} 7=2*9+b \\ 7=18+b \\ 7-18=b \\ b=-11 \end{gathered}`

Thus, the slope-intercept with inequality will be:

`y<2x-11`

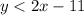
c. The standard form equation of a line is:

`ax+by=c`

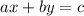
Let's use the third set of points (3,5) (5,4).

Start by finding the slope:

`m=(4-5)/(5-3)=(-1)/(2)=-0.5`

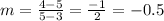
Now, you can start with the point-slope form and then convert it into the standard form:

`\begin{gathered} y-5\ge-0.5(x-3) \\ Apply\text{ the distributive property} \\ y-5\ge-0.5x+1.5 \\ y\ge-0.5x+1.5+5 \\ y\ge-0.5x+6.5 \\ 0.5x+y\ge6.5 \end{gathered}`

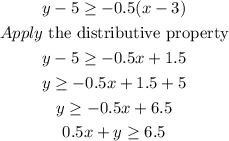
Where a=0.5, b=1 and c=6.5