Question

Sketch the graph of a function that has a local maximum value at x = a where f'(a) is undefined.

Answer 1

Derivative and Maximum Value of a Function

The critical points of a function are those where the first derivative is zero or does not exist.

Out of those points, we may find local maxima or minima or none of them.

One example of a function with a derivative that does not exist is:

`y=-x^{(2)/(3)}`

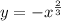
This function has a local maximum at x=0 where the derivative does not exist.

The graph of this function is shown below: