Question

A set of 12 data points is given above. Which of thefollowing is true of these data?

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Write the given data

`\lbrace14.9,21.1,21.2,8.4,14.5,5.9,7.6,10.0,4.8,3.2,28.7,29.5\rbrace`

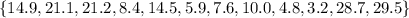
STEP 2: Find the mean ofthe data

STEP 3: Find the median

Hence, it can be seen here that the mean is larger than median.

STEP 4: Find the Interquartile range

`\begin{gathered} The\:interquartile\:range\:is\:the\:difference\:of\:the\:first\:and\:third\:quartiles \\ First\text{ Quartile}=6.75 \\ Third\text{ quartile}=21.15 \\ IQR=14.4 \end{gathered}`

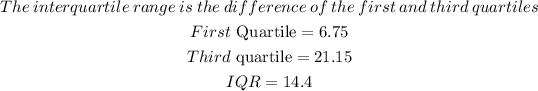
STEP 5: Find the standard deviation

`\begin{gathered} \mathrm{The\:standard\:deviation,\:}\sigma \left(X\right)\mathrm{,\:is\:the\:square\:root\:of\:the\:variance:\quad }\sigma \left(X\right)=\sqrt{\frac{\sum _(i=1)^n\left(x_i-\bar{x}\right)^2}{n-1}} \\ Standard\text{ deviation}=9.11836 \end{gathered}`

Hence, it can be seen from above that the interquartile range is larger than the standard deviation.

STEP 6: Find the range

`\begin{gathered} \mathrm{The\:range\:of\:the\:data\:is\:the\:difference\:between\:the\:maximum\:and\:the\:minimum\:of\:the\:data\:set} \\ Minimum=3.2 \\ Maximum=29.5 \\ Range=26.3 \end{gathered}`

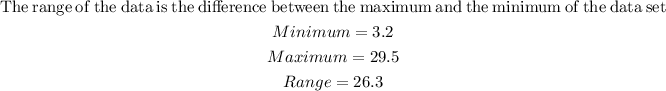
STEP 7: Fnd the variance

`\begin{gathered} \mathrm{The\:sample\:variance\:measures\:how\:much\:the\:data\:is\:spread\:out\:in\:the\:sample.} \\ \mathrm{For\:a\:data\:set\:}x_1,\:\ldots \:,\:x_n\mathrm{\:\left(n\:elements\right)\:with\:an\:average}\:\bar{x}\mathrm{,\:}Var\left(X\right)=\sum _(i=1)^n\frac{\left(x_i-\bar{x}\right)^2}{n-1} \\ Variance=83.14454 \end{gathered}`

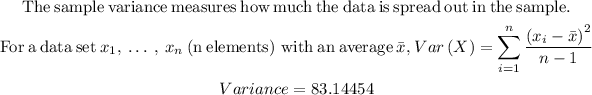
Hence, it can be seen that the range is not larger than the variance.

Therefore, the answer is I and II only.