Question

Please help me with 24For the following exercises, write the equation for the hyperbola in standard form if it is not already, and identify the vertices and foci, and write equations of asymptotes.

Answer 1

Given the equation,

`-9x^2+72x+16y^2+16y+4=0`

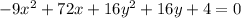
Complete squares as shown below,

`\begin{gathered} -9x^2+72x-a^2=-(9x^2-72x+a^2)=-9(x^2-8x+b^2) \\ \end{gathered}`

Thus,

`\begin{gathered} \Rightarrow-9x^2+72x-a^2=-9(x^{}-4)^2 \\ \Rightarrow a^2=16\cdot9=144\Rightarrow a=12 \\ \Rightarrow-9x^2+72x-144=-9(x^{}-4)^2 \end{gathered}`

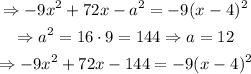
Similarly,

`\begin{gathered} 16y^2+16y=16(y^2+y) \\ \Rightarrow16(y+(1)/(2))^2=16(y^2+y+(1)/(4)) \end{gathered}`

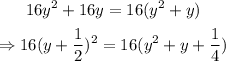
Therefore,

`\begin{gathered} -9x^2+72x+16y^2+16y+4=0 \\ \Rightarrow-9(x-4)^2+16(y+(1)/(2))^2+4=-144+4 \\ \Rightarrow-9(x-4)^2+16(y+(1)/(2))^2=-144 \end{gathered}`

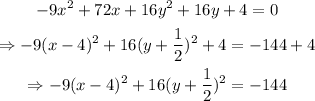
Finally, the standard form is.

`\begin{gathered} \Rightarrow-((x-4)^2)/(16)+((y+(1)/(2))^2)/(9)=-1 \\ \Rightarrow((x-4)^2)/(16)-((y+(1)/(2))^2)/(9)=1 \end{gathered}`

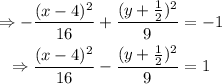
As for the vertices, foci, and asymptotes,

`\begin{gathered} c=\pm\sqrt[]{16+9}=\pm5 \\ \text{center:}(4,-(1)/(2)) \\ \Rightarrow\text{foci:}(4-5,-(1)/(2))_{},(4+5,-(1)/(2))_{} \\ \Rightarrow\text{foci:}(-1,-(1)/(2)),(9,-(1)/(2)) \end{gathered}`

Foci: (-1,-1/2), (9,-1/2)

Vertices

`\begin{gathered} \text{center:}(4,-(1)/(2)),\text{vertices:}(4\pm a,-(1)/(2)) \\ \text{vertices:}(4+4,-(1)/(2)),(4-4,-(1)/(2)) \\ \text{vertices:}(8,-(1)/(2)),(0,-(1)/(2)) \end{gathered}`

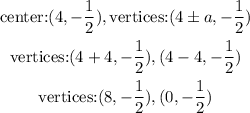
Vertices: (8,-1/2), (0,-1/2)

Asymptotes:

`\begin{gathered} y=\pm(3)/(4)(x-4)-(1)/(2) \\ \Rightarrow y=(3)/(4)x-(7)/(2) \\ \text{and} \\ y=-(3)/(4)x+(5)/(2) \end{gathered}`

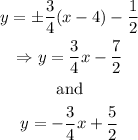
Asymptotes: y=3x/4-7/2 and y=-3x/4+5/2