so.. hmm notice the picture here
we know the length is 59 more than twice the width,
twice the width, 2 * w, or 2w
59 more than that
2w + 59
we also know that, whatever that is, the diagonal of it is,
2 more inches than that, or
(2w + 59) + 2
now.. use the pythagorean theorem
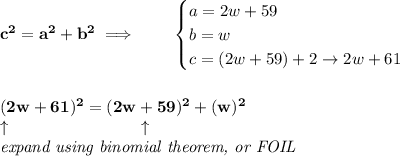
you'd end up with a quadratic, after simplifying, solve for "w"