Question

The number of microbes in a tissue sample is given by the functionN (t) = 34.8 + In(1 + 1.2t)where N(t) is the number of microbes (in thousands) in the sample after thours.a.) How many microbes are present initially?b.) How fast are the microbes increasing after 10 hours?

Answer 1

Step-by-step explanation

`N(t)=34.8+\ln (1+1.2t)`

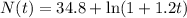
we have a function where the number of microbes ( N) depends on the time(t)

hence

Step 1

a.) How many microbes are present initially?

to know this, we need replace time I= t = zero, because it was "initially"

so

when t=0

replace.

`\begin{gathered} N(t)=34.8+\ln (1+1.2t) \\ N(0)=34.8+\ln (1+1.2\cdot0) \\ N(0)=34.8+\ln (1) \\ N(0)=34.8+0 \\ N(0)=34.8 \end{gathered}`

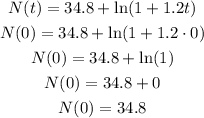
so, initially there were 34.8 microbes

Step 2

b)How fast are the microbes increasing after 10 hours?

to know this, let t=10

so

`\begin{gathered} N(t)=34.8+\ln (1+1.2t) \\ N(10)=34.8+\ln (1+1.2\cdot10) \\ N(10)=34.8+\ln (1+12) \\ N(10)=34.8+\ln (13) \\ N(10)=34.8+2.56 \\ N(10)=37.36 \end{gathered}`

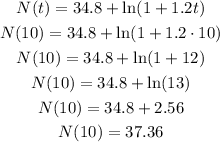
therefore , after 10 hours the number of microbes is 37.36

I hope this helps you