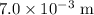Question

The wiring in a house must be thick enough so it does not become so hot as to start a fire.part aWhat diameter must a copper wire be if it is to carry a maximum current of 34 A and produce no more than 1.6 W of heat per meter of length?

Answer 1

Given:

The maximum current in the circuit is,

`i=34\text{ A}`

The power per length is,

`(P)/(l)=1.6\text{ W/m}`

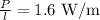
To find:

The diameter of the copper wire

Step-by-step explanation:

The power (P) produced by current i, through a copper wire of resistance R and length l is given by,

`\begin{gathered} Pl=i^2R \\ (R)/(l)=(P)/(i^2) \\ (R)/(l)=(1.6)/(34*34) \end{gathered}`

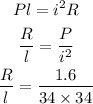
Now,

`\begin{gathered} R=(\rho l)/(A) \\ R=(\rho l)/(\pi r^2) \end{gathered}`

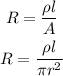
The resistivity of copper is,

`\rho=1.72*10^(-8)\text{ ohm.m}`

So, we can write,

`\begin{gathered} (R)/(l)=(\rho)/(\pi r^2) \\ (1.6)/(34*34)=(1.72*10^(-8))/(\pi r^2) \\ r^2=(1.72*10^(-8)*34*34)/(1.6) \\ r=3.5*10^(-3)\text{ m} \\ diamer\text{ is,} \\ 2r=7.0*10^(-3)\text{ m} \end{gathered}`

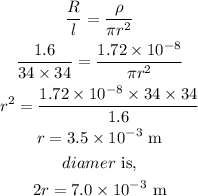
Hence, the diameter is,

`7.0*10^(-3)\text{ m}`