Question

John sells plain cakes for $8 and decorated cakes for $12. On a particular day, John started with a total of 100 cakes, and sold all but 4. His sales that day totaled $800.He sold ___plain cakes and ____decorated cakes that day.

Answer 1

INFORMATION:

We know that:

- John sells plain cakes for $8 and decorated cakes for $12.

- On a particular day, John started with a total of 100 cakes, and sold all but 4.

- His sales that day totaled $800.

And we must find the number of plain cakes and decorated cakes that he sold that day.

STEP BY STEP EXPLANATION:

To find them, we can represent the situation using a system of equations

`\begin{cases}x+y={100-4...(1)} \\ 8x+12y={800...(2)}\end{cases}`

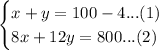
Where, x represents the number of plain cakes that he sold and y represents the number of decorated cakes that he sold.

Now, we must solve the system:

1. We must multiply the equation (1) by -8

`\begin{gathered} -8(x+y)=-8(100-4) \\ -8x-8y=-768...(3) \end{gathered}`

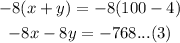
2. We must add equations (2) and (3)

`\begin{gathered} 8x+12y=800 \\ -8x-8y=-768 \\ ---------- \\ 0x+4y=32 \\ \text{ Simplifying, } \\ 4y=32...(4) \end{gathered}`

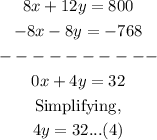
3. We must solve equation (4) for y

`\begin{gathered} 4y=32 \\ y=(32)/(4) \\ y=8 \end{gathered}`

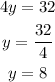
4. We must replace the value of y in equation (1) and then solve it for x

`\begin{gathered} x+8=100-4 \\ x=100-4-8 \\ x=88 \end{gathered}`

So, we found that x = 88 and y = 8.

Finally, John sold 88 plain cakes and 8 decorated cakes.

ANSWER:

He sold 88 plain cakes and 8 decorated cakes that day.