Question

Which operation results in a binomial?+(3y6 + 4)(9y12 - 12y6 + 16)ResetNextntum. All rights reserved.

Answer 1

Multiplication

`(3y^(6) + 4) . (9y^(12) -12y^(6) +16)`

`(9y^(12).3y^(6) -12y^(6).3y^(6) +16.3y^(6)) + (9y^(12).4 -12y^(6) .4 +16.4)`

Now, simplify each term:

`26y^(18) - 36y^(12) + 48y^(6) + 36y^(12) - 48y^(6) + 64`

`26y^(18) + 64`

Answer 2

Explanations:

According to the question, we need to determine which of the signs will fit in that will make the expression a binomial.

In simple terms, a binomial is a two-term algebraic expression that contains variable, coefficient, exponents, and constant.

We need to determine the required sign by using the trial and error method.

Using the positive sign (+) first, we will have:

`\begin{gathered} =\mleft(3y^6+4\mright)+(9y^(12)-12y^6+16) \\ =3y^6+4+9y^(12)-12y^6+16 \\ =3y^6-12y^6+4+9y^(12)+16 \\ =-9y^6+9y^(12)+20 \end{gathered}`

Using the product sign, this will be expressed as:

`\begin{gathered} (3y^6+4)\cdot(9y^(12)-12y^6+16) \\ (3y^6+4)\cdot\lbrack(3y^6)^2-(3y^6)(4)^{}+4^2)\rbrack \end{gathered}`

According to the sum of two cubes;

`a^3+b^3=\mleft(a+b\mright)•(a^2-ab+b^2)`

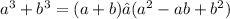
Comparing this with the expression above, we will see that a = 3y^6 and

b = 4. This means that the resulting expression above can be written as a sum of two cubes to have;

`\begin{gathered} (3y^6+4)\cdot\lbrack(3y^6)^2-(3y^6)(4)^{}+4^2)\rbrack^{} \\ =(3y^6)^3-4(3y^6)^2+4(3y^6)^2+16(3y^6)+4(3y^6)^2-16(3y^6)+4^3 \\ \end{gathered}`

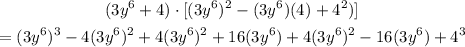
Collect the like terms: