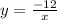Question

Write an equation for the inverse variation represented by the table.x -3, -1, 1/2, 2/3y 4, 12, -24, -18

Answer 1

By definition, Inverse variation equations have the following form:

`y=(k)/(x)`

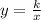
Where "k" is the Constant of variation.

Given the values shown in the table, you can find the value of "k":

- Choose a point from the table. This could be:

`(-3,4)`

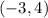
Notice that:

`\begin{gathered} x=-3 \\ y=4 \end{gathered}`

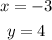
- Substitute these values into the equation and solve for "k":

`\begin{gathered} 4=(k)/(-3) \\ \\ (4)(-3)=k \\ k=-12 \end{gathered}`

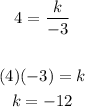
Knowing the Constant of variation, you can write the following equation:

`y=(-12)/(x)`

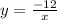
The answer is:

`y=(-12)/(x)`