Question

Find the slope of the graph of the function at the given point.

Answer 1

Step-by-step explanation:

Consider the following function:

`f(x)=\text{ }\tan(x)\text{ cot\lparen x\rparen}`

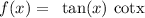
First, let's find the derivative of this function. For this, we will apply the product rule for derivatives:

`(df(x))/(dx)=\tan(x)\cdot(d)/(dx)\text{ cot\lparen x\rparen + }(d)/(dx)\text{ tan\lparen x\rparen }\cdot\text{ cot\lparen x\rparen}`

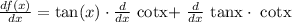
this is equivalent to:

`(df(x))/(dx)=\tan(x)\cdot(\text{ - csc}^2\text{\lparen x\rparen})\text{+ \lparen sec}^2(x)\text{\rparen}\cdot\text{ cot\lparen x\rparen}`

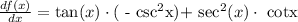
or

`(df(x))/(dx)=\text{ -}\tan(x)\cdot\text{ csc}^2\text{\lparen x\rparen+ sec}^2(x)\cdot\text{ cot\lparen x\rparen}`

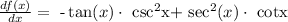
now, this is equivalent to:

`(df(x))/(dx)=\text{ -2 csc \lparen2x\rparen + 2 csc\lparen2x\rparen = 0}`

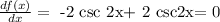
thus,

`(df(x))/(dx)=0`

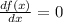
Now, to find the slope of the function f(x) at the point (x,y) = (1,1), lug the x-coordinate of the given point into the derivative (this is the slope of the function at the point):

`(df(1))/(dx)=0`

Notice that this slope matches the slope found on the graph of the function f(x), because horizontal lines have a slope 0:

We can conclude that the correct answer is:

Answer:

The slope of the graph f(x) at the point (1,1) is

`0`