Question

how many term has G.p whose 2nd term is 1/2 and common ratio and the last term are 1/4and1/128respestively

Answer 1

The geometric progression has the form:

`\mleft\lbrace a,ar,ar^2,ar^3,\ldots,ar^n\mright\rbrace`

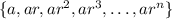
We have the information about the second term, a*r:

`ar=(1)/(2)`

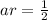
We know that the common ratio is

`r=(1)/(4)`

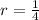
So from this information we can get the coefficient a:

`\begin{gathered} ar=(1)/(2) \\ a\cdot(1)/(4)=(1)/(2) \\ a=(4)/(2)=2 \end{gathered}`

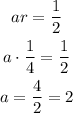
And we also know that the last term is 1/128, that is

`ar^n=(1)/(128)`

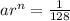
From this one we can find n:

`\begin{gathered} 2\cdot((1)/(4))^n=(1)/(128) \\ ((1)/(4))^n=(1)/(128\cdot2) \end{gathered}`

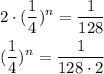
We can apply the property of the logarithm of power to get n:

`\begin{gathered} \log (((1)/(4))^n)=\log ((1)/(256)) \\ n\cdot\log ((1)/(4))^{}=\log ((1)/(256)) \\ n=(\log ((1)/(256)))/(\log ((1)/(4))) \\ n=4 \end{gathered}`

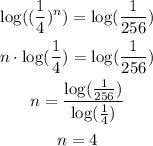
Be careful, because n is not the number of terms. The number of terms is n+1, so the G.P. has 5 terms