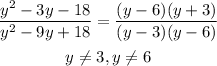Question

Reduce the rational expression to lowest terms. If it is already in lowest terms, enter the expression in the answer box. Also, specify any restrictions on the variable.y²-3y - 18/y²-9y + 18Rational expression in lowest terms:Variable restrictions for the original expression: y

Answer 1

Given: The expression below

`(y^2-3y-18)/(y^2-9y+18)`

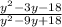
To Determine: The lowest term of the given rational fraction

Solution

Let simplify both the numerator and the denominator

`\begin{gathered} Numerator:y^2-3y-18 \\ y^2-3y-18=y^2-6y+3y-18 \\ y^2-3y-18=y(y-6)+3(y-6) \\ y^2-3y-18=(y-6)(y+3) \end{gathered}`
`\begin{gathered} Denominator:y^2-9y+18 \\ y^2-9y+18=y^2-3y-6y+18 \\ y^2-9y+18=y(y-3)-6(y-3) \\ y^2-9y+18=(y-3)(y-6) \end{gathered}`

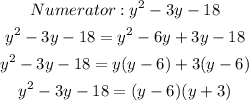
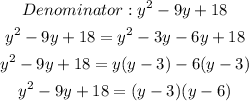
Therefore

`\begin{gathered} (y^2-3y-18)/(y^2-9y+18)=((y-6)(y+3))/((y-3)(y-6)) \\ y-6-is\text{ common} \\ (y^(2)-3y-18)/(y^(2)-9y+18)=((y-6)(y+3))/((y-3)(y-6)) \\ (y^(2)-3y-18)/(y^(2)-9y+18)=(y+3)/(y-3) \end{gathered}`

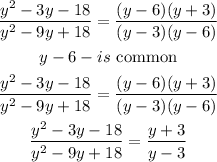
Hence, the rational expression in its lowest term is

`(y+3)/(y-3)`

The variable for the original expression is as given as

`\begin{gathered} (y^(2)-3y-18)/(y^(2)-9y+18)=((y-6)(y+3))/((y-3)(y-6)) \\ y\\e3,y\\e6 \end{gathered}`