The general equation of a line passing through two points (xb₁,y₁)Pxb₂,y₂) is expressed as
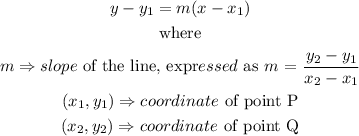
Given that the coordinates of the two points are (-2, -3) and (3, -5), we have

Step 1:
Evaluate the slope o the line.
The slope is thus evaluated as
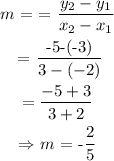
Step 2:
Substitute the values of x₁,
Thus, we have
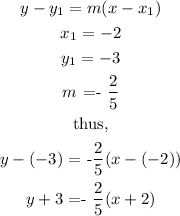
Step 3:
Make .
![\begin{gathered} y+3\text{ =- }(2)/(5)(x+2) \\ \text{Multiply both sides of the equation by 5 } \\ 5(y+3)\text{ = -2(x+2)} \\ \text{open brackets} \\ 5y\text{ + 15 =- 2x - 4} \\ \Rightarrow5y\text{ =- 2x - 4 -15} \\ 5y\text{ = -2x-1}9 \\ \text{divide both sides of the equation by the coefficient of y, which is 5.} \\ \text{thus,} \\ (5y)/(5)=\frac{-\text{2x-1}9}{5} \\ \Rightarrow y\text{ =- }(2)/(5)x\text{ - }(19)/(5) \end{gathered}]()
Hence, the equation of the line is
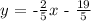
y₁ and m into the general equation of the line.