Answer:
The answer is 1 i.e the inverse function will be 1 when x=19
Explanation:
Given
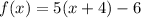
we have to solve for inverse function
Step 1: Replace f(x) with y.
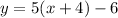
Step 2: Now replace all x with y and all y with x, we get
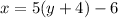
Step 3: Solve for y
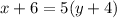
⇒
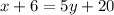
⇒
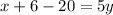
⇒
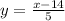
Step4: Replace y by
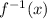
gives
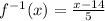
When x=19 (given)
⇒
