Question

in the figure below, RTS is an isosceles triangle with sides SR=RT, TVU is an equilateral triangle, WT is the bisected of angle STV, points S, T, and U are collinear, and c= 40 degrees.I'm completely lost and have to answer for a, b+c, f, b+f, a+d, e+g

Answer 1

Step 1: Concept

Triangle SRT is an isosceles triangle with equal base angles a = b

Triangle TUV is an equilateral triangle with all angles equal: g = d = h

Step 2: Apply sum of angles in a triangle theorem to find angle a and b.

`\begin{gathered} a+b+c=180^o \\ c=40^o \\ \text{Let a = b = x} \\ \text{Therefore} \\ x\text{ + x + 40 = 180} \\ 2x\text{ = 180 - 40} \\ 2x\text{ = 140} \\ x\text{ = }(140)/(2) \\ x\text{ = 70} \\ a\text{ = 70 and b = 70} \end{gathered}`

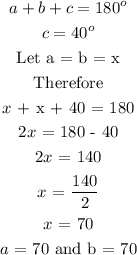
Step 3:

2) a = 70

3) b + c = 70 + 40 = 110

Step 4:

Since WT is a bisector of angle STV,

Angle f = e = x

b + f + e = 180 sum of angles on a straight line.

b = 70

70 + x + x = 180

2x = 180 - 70

2x = 110

x = 110/2

x = 55

Hence, f = 55

4) f = 55

5) f + b = 55 + 70 = 125

Step 5:

Since triangle TUV is an equilateral triangle, angle g = h = d = 60

g = 60

h = 60

d = 60

6) Angle a + d = 70 + 60 = 130

7) e + g = 55 + 60 = 115