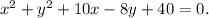Question

A circle is centered at c(-5,4)and has a radius of 2. Find the equation of a concentric circle with half the radius.

Answer 1

the answer to this is (x+5)^2+(y-4)^2=1

Answer 2

Answer: The required equation of the concentric circle is

`x^2+y^2+10x-8y+40=0.`

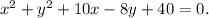
Step-by-step explanation: Given that a circle is centered at the point C(-5,4) and has a radius of 2 units.

We are to find the equation of a concentric circle with half the radius.

We know that

the standard equation of a circle with center at the point (h, k) and radius 'r' units is given by

`(x-h)^2+(y-k)^2=r^2~~~~~~~~~~~~~~~~~~~~~~~(i)`

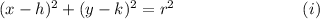
Since the centers of two concentric circles are same, so the center of the circle will be

center, (h, k) = (-5, 4) and radius is equal to half of 2 units.

So, radius of the concentric circle will be

`r=(2)/(2)=1~\textup{unit}.`

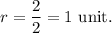
Therefore, the equation of the concentric circle is

`(x-h)^2+(y-k)^2=r^2\\\\\Rightarrow (x-(-5))^2+(y-4)^2=1^2\\\\\Rightarrow (x+5)^2+(y-4)^2=1\\\\\Rightarrow x^2+10x+25+y^2-8y+16=1\\\\\Rightarrow x^2+y^2+10x-8y+40=0.`

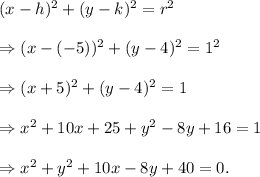
Thus, the required equation of the concentric circle is

`x^2+y^2+10x-8y+40=0.`