Question

Answer the questions below about the quadratic function.g(×)=2×^2-12×+19Does the function have a minimum or maximum? minimum or maximum what is the functions minimum or maximum value?Where does the minimum or maximum value occur?x=?

Answer 1

Given the function:

`g(x)=2x^2-12x+19`

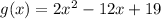
Let's determine if the function has a minimum or maximum.

The minimum and maximum of a function are the smallest and largest value of a function in a given range or domain

The given function has a minimum.

Apply the general equation of a quadratic function:

`y=ax^2+bx+c`

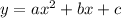
To find the minimum value, apply the formula:

`x=-(b)/(2a)`

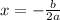
Where:

b = -12

a = 2

Thus, we have:

`\begin{gathered} x=-(-12)/(2(2)) \\ \\ x=-(-12)/(4) \\ \\ x=3 \end{gathered}`

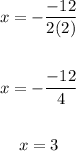
To find the function's minimum value, find f(3).

Substitute 3 for x in the function and evaluate:

`\begin{gathered} f(x)=2x^2-12x+19 \\ \\ f(3)=2(3)^2-12(3)+19 \\ \\ f(3)=2(9)-36+19 \\ \\ f(3)=18-36+19 \\ \\ f(3)=1 \end{gathered}`

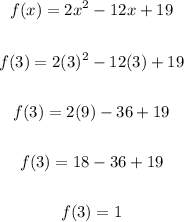
Therefore, the function's minimum value is 1

Therefore, the functions minimum value occurs at:

x = 3

ANSWER:

• The function has a minimum

• Minimum value: 1

• The minimum occurs at: x = 3