Question

How do find the domain and range of
`(1)/(√(x-8))`?

Answer 1

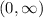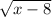
`√(x-8)` is undefined if the argument
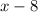
`x-8` is negative, so you first need to require that


`x-8\ge0\implies x\ge8`

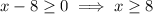
We're not done yet, though, because
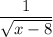
`\frac1{√(x-8)}` still doesn't exist when
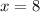
`x=8`, so we remove this from the domain and we're left with
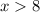
`x>8`, or in interval notation,
`(8,\infty)`

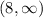
To find the range, consider the limits of the function as you approach either endpoint of the domain.


`\displaystyle\lim_(x\to8^+)\frac1{√(x-8)}=+\infty`

`\displaystyle\lim_(x\to\infty)\frac1{√(x-8)}=0`

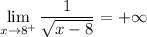
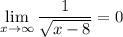
Since
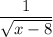
`\frac1{√(x-8)}` is positive everywhere, the range is
`(0,\infty)`