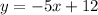Answer:
The equation of the line is
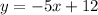
Explanation:
Step 1
Find the slope of the given line
we have

Isolate the variable y
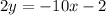

The slope of the given line is
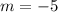
Step 2
Find the slope of the line that is parallel to the given line
we know that
if two lines are parallel, then their slopes are the same
so
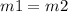
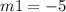 ----> slope of the given line
----> slope of the given line
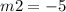 ----> slope of the line parallel to the given line
----> slope of the line parallel to the given line
Step 3
Find the equation of the line parallel to the given line that passes through the point

we know that
the equation of the line in slope-intercept form is equal to
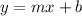
where
m is the slope of the line
b is the y-intercept (value of y when the value of x is equal to zero)
In this problem we have
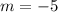
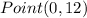 -------> this is the y-intercept
-------> this is the y-intercept
so
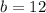
substitute
the equation of the line is