Question

Suppose a basketball player has made 359 out of 449 free throws. If the player makes the next 3 free throws, I will pay you $39. Otherwise you pay me $43.

Step 2 of 2 : If you played this game 623 times how much would you expect to win or lose?

Answer 1

Answer: expect to lose 679.07 dollars

==========================================================

Step-by-step explanation:

Assuming each free throw is independent of any other, the probability of making the next free throw is 359/449

The probability of making 3 in a row is (359/449)^3 = 0.511145 approximately which represents the probability of earning the $39

That must mean 1-0.511145 = 0.488855 is the approximate probability of losing $43

Let's make a table of outcomes and their associated probabilities.

X = amount of money the player earns (the person shooting the free throws)

`\begin{array}c \cline{1-2}\text{X} & \text{P(X)}\\\cline{1-2}39 & 0.511145\\\cline{1-2}-43 & 0.488855\\\cline{1-2}\end{array}`

Then from here we'll multiply each X and P(X) value for each separate row.

Example: 39*0.511145 = 19.934655

Let's form a third column of these products

`\begin{array}c \cline{1-3}\text{X} & \text{P(X)} & \text{X}*\text{P(X)}\\\cline{1-3}39 & 0.511145 & 19.934655\\\cline{1-3}-43 & 0.488855 & -21.020765\\\cline{1-3}\end{array}`

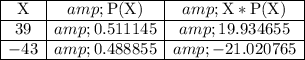
Add up everything in the X*P(X) column and you should get roughly -1.08611 which rounds to -1.09

The player expects, on average, to lose about $1.09 each time they play this game. Playing 623 times means they should expect to lose 623*1.09 = 679.07 dollars

Of course, given the nature of this random process, it's not a guarantee they will lose this amount. This is just the average of many attempts.