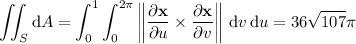I assume the equation of the plane is
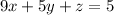
and the cylinder has equation
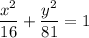
.
I don't know what techniques are available to you, so I'll resort to (in my opinion) the most reliable: surface integration.
The surface of intersection
is an ellipse in three dimensional space which can be parameterized by
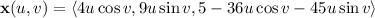
, with
![u\in[0,1]](https://img.qammunity.org/2018/formulas/mathematics/high-school/r96tnw02gcggywffdvqf3f0hg9ziu7rtzi.png)
and
![v\in[0,2\pi]](https://img.qammunity.org/2018/formulas/mathematics/high-school/uqpdql9evuj6e7qv4lhr3wk7yaeaeq6prc.png)
.
The area is then given by the integral