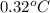Answer : The freezing point of a solution is,
Explanation :
First we have to calculate the Van't Hoff factor (i) for
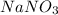 .
.
The dissociation of
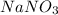 will be,
will be,
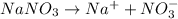
So, Van't Hoff factor = Number of solute particles =
 = 1 + 1 = 2
= 1 + 1 = 2
Now we have to calculate the freezing point of a solution.
Formula used for lowering in freezing point :
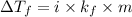
or,
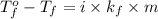
where,
 = change in freezing point
= change in freezing point
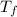 = temperature of solution = ?
= temperature of solution = ?
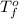 = temperature of pure water =
= temperature of pure water =
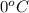
 = freezing point constant =
= freezing point constant =

m = molality = 0.08500 m
i = Van't Hoff factor = 2
Now put all the given values in this formula, we get the freezing point of a solution.
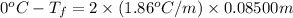

Therefore, the freezing point of a solution is,