Question

Find the quotient of the complex numbers

Answer 1

Answer:
`(2)/(9)\left(\cos\left((\pi)/(132)\right)+i*\sin\left((\pi)/(132)\right)\right)`

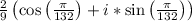
Note: refresh the page if the symbols aren't showing up correctly

If the text is too small, then check out the attached image for a bigger version of the same answer

The answer written out in keyboard form is (2/9)*(cos(pi/132)+i*sin(pi/132))

=======================================

Work Shown:

z1 = a*(cos(b) + i*sin(b))
z1 = 2*(cos(pi/11) + i*sin(pi/11))
here we see that a = 2 and b = pi/11

z2 = c*(cos(d) + i*sin(d))
z2 = 9*(cos(pi/12) + i*sin(pi/12))
here we see that c = 9 and d = pi/12
----------------

Now use the rule
If
z = a*(cos(b) + i*sin(b)) and w = c*(cos(d)+i*sin(d))
then
z/w = (a/c)*(cos(b-d)+i*sin(b-d))

We have
a = 2
b = pi/11
c = 9
d = pi/12

So...

z/w = (a/c)*(cos(b-d)+i*sin(b-d))
(z1)/(z2) = (a/c)*(cos(b-d)+i*sin(b-d))
(z1)/(z2) = (2/9)*(cos(pi/11-pi/12)+i*sin(pi/11-pi/12))
(z1)/(z2) = (2/9)*(cos(12pi/132-11pi/132)+i*sin(12pi/132-11pi/132))
(z1)/(z2) = (2/9)*(cos(pi/132)+i*sin(pi/132))
which is the answer in polar form