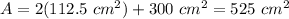Question

In an isosceles trapezoid the length of a diagonal is 25 cm and the length of an altitude is 15 cm. Find the area of the trapezoid.

Lots of points

Answer 1

Answer: The answer is 300 cm^2 I think.

Answer 2

The area of the trapezoid is
`525\ cm^(2)`

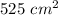
Explanation:

we know that

The area of a isosceles trapezoid is equal to the area of two isosceles right triangles plus the area of a rectangle

step 1

Find the area of the isosceles right triangle

Remember that

In a isosceles right triangle the height is equal to the base of the triangle

we have

`h=15\ cm`

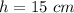
so

`b=15\ cm`

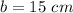
The area is equal to

`A=(1)/(2)(b)(h)`

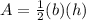
substitute the values

`A=(1)/(2)(15)(15)=112.5\ cm^(2)`

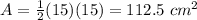
step 2

Find the area of the rectangle

The area of the rectangle is equal to

`A=LW`

we have

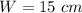`W=15\ cm` -----> is the height of the trapezoid

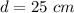`d=25\ cm` -----> the diagonal of the rectangle

Applying the Pythagoras Theorem

`25^(2)=L^(2)+15^(2)\\L^(2)=25^(2)-15^(2) \\ L^(2) =400\\L=20\ cm`

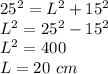
The area of the rectangle is

`A=(20)(15)=300\ cm^(2)`

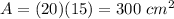
step 3

Find the area of the trapezoid

`A=2(112.5\ cm^(2))+300\ cm^(2)=525\ cm^(2)`