Question

Given the system below:

ƒ(x) = 2x
g(x) = 2x

Which value(s) of x make ƒ(x) = g(x) a true statement? If necessary, you may choose more than one answer.

0
1
2
3
4

Answer 1

if F(x) =g(x) =2x,
x can be any value

Answer 2

For
`f(x)=g(x)` we can take any value of x =0,1,2,3 etc

Explanation:

Given the system

f(x)=
`2x`

And g(x)=
`2x`

We have f(x)= g(x)=
`2x`

Therferore , the graph of two function is coincident line .

The solutions from graph of two functions are infinite because both functions are the same .

Therefore, the value of both functions are same at every value of x.

Therefore, we can choose more tha one value of x.

For examaple, at x=0

f(x)=0 and g(x)=0

At x=1 we get

f(x)=2

And g(x)=2

At x=3 we get

f(x) =
`3*2=6`

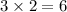
g(x)=
`3*2=6`

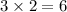
Therefore , we can see at every value of x the value of two functions are same.

Therefore, we can take x=0,1,2,3,4