Answer:
The function f(x) is stretched horizontally.
Explanation:
We are asked to determine how the graph of
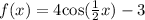 differ from the graph of
differ from the graph of
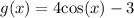 .
.
Let us recall transformation rules of functions.

If
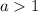 function compresses horizontally.
function compresses horizontally.
If
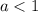 function stretches horizontally.
function stretches horizontally.
Upon looking at function
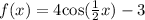 , we can see that the value of 'a' is less than 1 that is
, we can see that the value of 'a' is less than 1 that is
 , therefore, the function f(x) is stretched horizontally.
, therefore, the function f(x) is stretched horizontally.