Question

Graph the solution set l 2x + 4l + 2 <= 8

Answer 1

EXPLANATION :

From the problem, we have :

`\begin{gathered} \lvert{2x+4}\rvert+2\le8 \\ \text{ which can be simplified as :} \\ \lvert{2x+4}\rvert\le6 \end{gathered}`

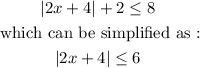
Note that in solving absolute values, the terms inside the absolute value sign can have inverse signs.

Case 1 : Positive

`\begin{gathered} 2x+4\le6 \\ 2x\le2 \\ x\le(2)/(2) \\ \\ x\le1 \end{gathered}`

Case 2 : Negative, the symbol will change since we are multiplying a negative number

`\begin{gathered} -(2x+4)\ge6 \\ -2x-4\ge6 \\ -2x\ge10 \\ x\ge(10)/(-2) \\ \\ x\ge-5 \end{gathered}`

So the solution is :

`\begin{gathered} x\le1\quad and\quad x\ge-5 \\ \text{ when written together :} \\ -5\le x\le1 \end{gathered}`

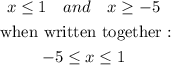
or in interval notation :

[-5, 1]

The graph of it will be :