Question

in right triangles ABC, angle A and B are complementary angles. the value of cos a is 5/13 is the value of sin B

Answer 1

we know that
In a right triangle
if A and B are complementary angles
cos A=sin B
therefore
if cos A=5/13
then sin B=5/13

the answer is
sin B=5/13

Answer 2

The value of sin B is 5/13.

Explanation:

In , Right triangles ABC:

∠A + ∠B = 90° (complimentary angles)

∠C = ?

In ΔABC:

∠A + ∠B + ∠C = 180° (angle sum property)

90° + ∠C = 180°

∠C= 180° - 90° = 90°

So, in right triangles ABC, angle 90° is at C.

According to trigonometric ratios:

`\cos \theta =(base)/(hypotenuse)`

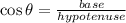
In right triangle ABC with base AC:

`\cos A=(5)/(13)=(AC)/(AB)`

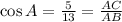
AC = 5. AB = 13

In right triangle ABC with base BC, then perpendicular becomes AC and hypotenuse is AB.

According to trigonometric ratios:

`\sin\theta =(perpendicular)/(hypotenuse)`

`\Sin B=(5)/(13)`

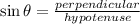
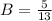
The value of sin B is 5/13.