Hey there!
First, you must find the common denominator of the equation.
To find the common denominator, first take a look at all the factors of each denominator:
x: x
x+4: x+4
6: 2*3
Next, because you do not have a common multiple in the denominators, you would multiply the denominators together to create one common multiple:
6(x)(x+4). This would be used to remove the fractions from the equation to make it easier to solve.
Now, multiply the common multiple 6(x)(x+4) to the entire equation:
(6(x)(x+4)) × (
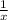
+
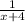
=
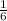
)
When you multiply the factor to the equation, the x in the common factor would cancel out the x in
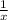
resulting in just 6(x+4).
The x+4 would cancel out the x+4 factor in
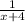
resulting in just 6x.
The 6 would cancel out the 6 in
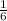
resulting in just x(x+4).
As a result, when you multiply the common factor 6(x)(x+4) to
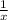
+
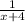
=
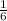
, you will get
(6(x+4))+ 6x = x(x+4)
Now, simplify the equation further:
(6(x+4))+ 6x = x(x+4)
6x+24+6x=x^2+4x (I have distributed the values in the parentheses)
12x+24=x^2 + 4x (I combined like terms on both sides)
0=x^2-8x-24 (I have moved all terms to one side so that the x values can be solved for using the quadratic formula)
Because this quadratic does not factor evenly, we must use the quadratic formula in order to find the exact x values:
x=
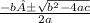
Your a value is 1, your b value is -8, and your c value is -24:
x=
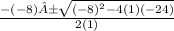
x=
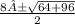
x=

x=
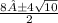
x=4±2

Therefore, your x values are 4+2

and 4-2
