Question

A volcano in the approximate shape of a cone has a diameter of 16 km and a height of 1.95 km. Which estimate best approximates the volume of the volcano? Use 3.14 for pi and round to nearest whole number. Remember to show all calculations!

Answer 1

Volume = 1/3 * pi * r^2 * h
= 1/3 * pi * 8^2 * 1.5 ( radius = 1/2 diameter = 1/2 * 16 = 8)
= 130.7 km^3 Answer

Answer 2

`130.624km^(3)`

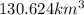
Explanation:

Given : A volcano in shape of a cone has a diameter of 16 km and a height of 1.95 km.

To Find : Volume of Volcano.

Solution :

Since we are given that volcano is in shape of cone .

So, Formula of volume of cone :
`(1)/(3) \pi r^(2)h`

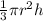
Height = 1.95 km.

Diameter = 16 km

Radius (r) = Diameter/2 = 16/2 =8 km

π = 3.14

Substituting all values in formula

Volume of volcano:

=
`(1)/(3) *3.14 (8)^(2)*1.95`

⇒
`(391.872)/(3)`

⇒
`130.624km^(3)`

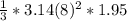
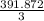
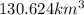
Thus , The volume of volcano is
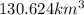`130.624km^(3)` i.e. 131 cubic km. nearest to whole number