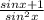Question

Cscx×(1+cscx)=(sinx+1/sin^2x)

Answer 1

We're going to verify this by working on the left side. We'll be using the reciprocal identity and some algebra skills.

cscx(1 + cscx) =
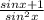
`(sinx + 1)/(sin^2x)` Go ahead and write the left side by itself.

cscx (1 + cscx) Distribute the cscx into the parentheses (mulitply)

cscx + csc²x Use the reciprocal identity to change cscx into
`(1)/(sinx)`


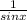
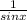
`(1)/(sinx)` + csc²x Use the same identity to change csc²x into
`(1)/(sin^2x)`


`(1)/(sinx)` +
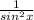
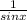
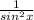
`(1)/(sin^2x)` Multiply
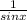
`(1)/(sinx)` by
`(sinx)/(sinx)` (it's the same as multiplying it by 1)


`(sinx)/(sin^2x)` +
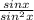
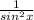
`(1)/(sin^2x)` Combine the numerators


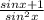
`(sinx + 1)/(sin^2x)` =
`(sinx + 1)/(sin^2x)`