Question

A) Plot the graph of y = 2^x+2 for-4 ≤ x ≤ 4

b) Draw the asymptote.
c) Write the equation of the asymptote.
I need help with question c only​

Answer 1

y = 2

Explanation:

A horizontal asymptote is a horizontal line that the graph of a function approaches as x approaches ±∞.

Mathematically we write this as

`f(x) \rightarrow a \text{ as } x \rightarrow \infty \text{ or } x \rightarrow -\infty`

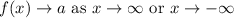
A vertical asymptote at some constant a is where the function approaches ±∞.

Mathematically

`f(x) \rightarrow \infty \text { or } f(x) \rightarrow -\infty \text{ as } x \rightarrow a`

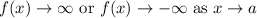
The given function y = 2ˣ + 2 is an exponential function and exponential functions do not have a vertical asymptote

The horizontal asymptote can be found by determining what the function limit is as
`x \rightarrow \pm \infty`

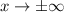
As
`x \rightarrow +\infty` the function
`\rightarrow \infty`

As
`x \rightarrow -\infty` ,
`2^x \rightarrow 0\\\\\\2^x + 2 \rightarrow 2\\\\`

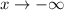So the equation for the horizontal asymptote is y = 2

The graph shows that at y = 2, the function is parallel to the line but does not touch the line