Answer:
Option D is correct
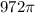 is the volume, in cubic units, of this sphere
is the volume, in cubic units, of this sphere
Explanation:
Surface area of sphere(S) and volume of sphere (V) is given by:
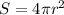
 .....[1]
.....[1]
As per the statement:
Suppose the surface area of a sphere is 324π square units
⇒S = 324π square units
then;
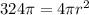
Divide both side by
 we have;
we have;
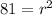
or
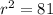
⇒
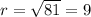 units
units
We have to find the volume, in cubic units, of this sphere.
Substitute the given value in [1] we have;
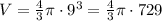
Simplify:
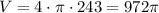 cubic units
cubic units
Therefore,
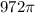 is the volume, in cubic units, of this sphere
is the volume, in cubic units, of this sphere