Question

Given: KLMN is a trapezoid, KF = 1, MF ║ LK , altitude −h. Area of KLMF = AFMN. Find: KN

Answer 1

it's 3 units due to the fact that KF is = to 1 and you need it to have the same area of the triangle. So I got 3

Answer 2

The measure of KN is 3 units.

Explanation:

Given information: KLMN is a trapezoid, KF = 1, MF ║ LK , altitude is h. Area of KLMF = Area of FMN.

The area of parallelogram is

`A=base * height`

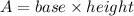
Area of parallelogram KLMF is

`A_1=KF * h`

`A_1=1 * h`

`A_1=h`

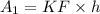
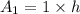
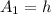
Area of a triangle is

`A=(1)/(2)* base* height`

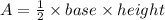
Area of triangle FMN is

`A_2=(1)/(2)* FN* h`

`A_2=(h(FN))/(2)`

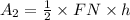
It is given that Area of KLMF = Area of FMN.

`A_1=A_2`

`h=(h(FN))/(2)`

`(2h)/(h)=FN`

`2=FN`

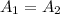
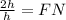
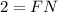
The length of FN is 2 units.

The length of KN is

`KN=KF+FN=1+2=3`

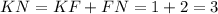
Therefore, the measure of KN is 3 units.