Answer:
The statement is slope of WX and YW are opposite reciprocals
Explanation:
Given the graph in which triangle is shown
we have to choose the statement that verifies that triangle WXY is a right triangle.
Coordinates of W, X and Y are
W=(-2,3)
X=(2,1.4)
Y=(-4,-2)
To verify the triangle WXY a right triangle, we have to find the slope of these lines
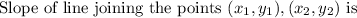
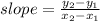
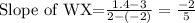

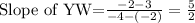
We see slope of WX and YW are opposite reciprocals that means these two lines are perpendicular which verifies that triangle WXY is a right triangle.
Hence, option 1 is correct