Question

According to the Rational Root Theorem, what are all the potential rational roots of f(x) = 9x4 – 2x2 – 3x + 4?

Answer 1

Option 1.

Explanation:

According to rational root theorem, all the potential rational roots of a function f(x) is defined as

`roots=(p)/(q)`

where, p is a factor of constant term and q is the factor of leading coefficient.

The given function is

`f(x)=9x^4-2x^2-3x+4`

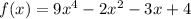
Here, leading coefficient is 9 and factors of 9 are ±1, ±3, ±9.

Constant term is 4 and factors of 4 are ±1, ±2, ±4.

All the potential rational roots of f(x) are

`\pm (1)/(9),\pm (2)/(9),\pm (4)/(9),\pm (1)/(3),\pm (2)/(3),\pm (4)/(3),\pm 1,\pm 2,\pm 4`

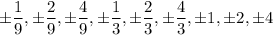
Therefore, option 1 is correct.

Answer 2

the answer is the first choice - see picture for solution: