Answer:
Using the tangent ratio:
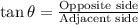
As per the given diagram:
In right angle triangle ACB:
Side BC = y units and Side AC =5 units
Apply the tangent ratio:

Opposite side = BC = y units and Adjacent side = AC = 5 units
Substitute the given values we have;
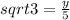
Multiply both sides by 5 we have;
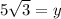
or
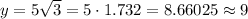
Therefore, the exact value of y is, 9 units