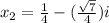Question

What are the solutions to the equation

Answer 1

C.

`x_1=(1)/(4)+((√(7))/(4))i` and
`x_2=(1)/(4)-((√(7) )/(4))i`

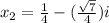
Explanation:

You have the quadratic function
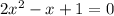`2x^2-x+1=0` to find the solutions for this equation we are going to use Bhaskara's Formula.

For the quadratic functions
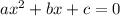`ax^2+bx+c=0` with
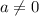`a\\eq 0` the Bhaskara's Formula is:

`x_1=(-b+√(b^2-4.a.c) )/(2.a)`

`x_2=(-b-√(b^2-4.a.c) )/(2.a)`

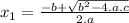
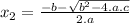
It usually has two solutions.

Then we have
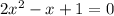`2x^2-x+1=0` where a=2, b=-1 and c=1. Applying the formula:

`x_1=(-b+√(b^2-4.a.c) )/(2.a)\\\\x_1=(-(-1)+√((-1)^2-4.2.1) )/(2.2)\\\\x_1=(1+√(1-8) )/(4)\\\\x_1=(1+√(-7) )/(4)\\\\x_1=(1+√((-1).7) )/(4)\\x_1=(1+√(-1).√(7))/(4)`

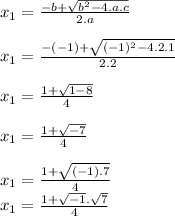
Observation:
`√(-1)=i`

`x_1=(1+√(-1).√(7))/(4)\\\\x_1=(1+i.√(7))/(4)\\\\x_1=(1)/(4)+((√(7))/(4))i`

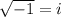
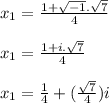
And,

`x_2=(-b-√(b^2-4.a.c) )/(2.a)\\\\x_2=(-(-1)-√((-1)^2-4.2.1) )/(2.2)\\\\x_2=(1-i.√(7) )/(4)\\\\x_2=(1)/(4)-((√(7))/(4))i`

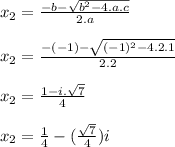
Then the correct answer is option C.

`x_1=(1)/(4)+((√(7))/(4))i` and
`x_2=(1)/(4)-((√(7) )/(4))i`